Synthesis, characterization, and design of molecular materials, especially molecular conductors (including superconductors), have been undertaken. Molecular conductors exhibit a variety of physical properties which can be systematically understood on the basis of "simple" and "clear" electronic structures. From a chemical point of view, the most fascinating character of the molecular conductor is its "designability", that is, we can finely control solid state properties with chemical modifications of the molecule. The newly synthesized materials are characterized by the X-ray diffraction method and physical measurements (electrical conductivity...etc.). The electronic structure is investigated by the band structure calculation. All these results are devoted to the design of new molecular materials.
- Magnetic-torque study on antiferromagnetic states of organic triangular-lattice systems, X[Pd(dmit)2]2 (X = Me4P and Me4Sb) (Isono, Cui, Kato; Sugiura, Terashima, Uji)
- Electron spin resonance of the molecular conductors X[Pd(dmit)2]2 which have triangular lattice (Oshima, Kim, Kato)
- Magnetic correlation madiated by spin-orbit coupling in (Cation)[Pt(dmit)2]2 (Fujiyama, Kato)
- Metallic state of a single-component molecular crystal [Au(hfdt)2] under high pressure (Cui, Kawasugi, Kato; Tsumuraya)
- Simultaneous enhancement of conductivity and Seebeck coefficient in an organic Mott transistor (Kawasugi, Sato, Kato; Seki, Edagawa, Pu, Takenobu, Yunoki, Yamamoto)
- Exploring low-temperature carrier transport around Mott transition using solid-gate-type field-effect transistor of a molecular conductor (Sato, Kawasugi, Kato; Yamamoto)
- Antiferromagnetic insulating phase of λ-(BETS)2FeCl4 studied by electron spin resonance (Oshima, Lee, Cui, Kato)
- Control of Dirac mass by hydrostatic pressure (Kawasugi, Kato; Tajima, Suda, H. Yamamoto)
- Transports properties through the Mott transition in Pd(dmit)2 based compounds (Abdel-J, Kato; Watanabe, Tajima, Ishii)
- Magnetic-torque study on antiferromagnetic states of organic triangular-lattice systems, X[Pd(dmit)2]2 (X = Me4P and Me4Sb)
- Electron Spin Resonance of the Molecular Conductors X[Pd(dmit)2]2 which have triangular lattice
- Magnetic correlation madiated by spin-orbit coupling in (Cation)[Pt(dmit)2]2
- Metallic state of a single-component molecular crystal [Au(hfdt)2] under high pressure
- Simultaneous enhancement of conductivity and Seebeck coefficient in an organic Mott transistor
- Exploring low-temperature carrier transport around Mott transition using solid-gate-type field-effect transistor of a molecular conductor
- Antiferromagnetic insulating phase of λ-(BETS)2FeCl4 studied by electron spin resonance
- Control of Dirac mass by hydrostatic pressure
- Transports properties through the Mott transition in Pd(dmit)2 based compounds
Assign: Isono, Cui, Kato; Sugiura, Terashima, Uji
In general, antiferromagnetically interacting spins form a Néel order at sufficiently low temperatures. By contrast, spins on a triangular lattice cannot satisfy the antiparallel paring on all bonds because of competing antiferromagnetic (AF) interactions (spin frustration); the Néel state is destabilized by the frustration. Organic triangular-lattice antiferromagnets, X[Pd(dmit)2]2, were first synthesized in Kato Lab. A characteristic feature of this system is that the degree of frustration can be precisely controlled by chemical substitution of a cation X+, leading to a variety of ground states such as AF, quantum spin-liquid (QSL), and non-magnetic charge-ordered states [1]. Among them, the QSL state of X = EtMe3Sb was intensively studied, and characteristic properties of it were revealed: the presence of gapless magnetic excitations at very low temperatures [2], and high spin thermal conductivity comparable to that of metals [3]. In this context, recently, SQUID magnetization measurements on AF X[Pd(dmit)2]2 have found that the uniaxial anisotropy of the AF state disappears when a cation X is substituted from Me4P to Me4Sb, that is the system gets closer to the QSL region [4]. This result suggests that the magnetic structure is changed in the AF state located near the QSL region.
In the present work, we performed torque magnetometry on the Me4P and Me4Sb salts to precisely probe the magnetic anisotropy of the AF states. Figure 1a and b show the magnetic-field dependence of the magnetic torque at T = 1.5 K for X = Me4P and Me4Sb, respectively. Here, the magnetic field is applied within the crystallographic ac* plane (Fig. 1c). The magnetic torque exhibits a peak structure near θ = 40 ° for the Me4P salt and near θ = 30 ° for the Me4Sb salt, and then the peak changes its sign in higher field angles. This behavior is a hallmark of a spin-flop transition [5]; not only the Me4P salt, but also the Me4Sb salt is a uniaxial antiferromagnet. The spin-flop field of the Me4Sb salt (~ 1.1 T) is half of the Me4P salt (~ 2.2 T). In usual uniaxial antiferromagnets, the spin-flop field HSF is described as HSF = [2D/(χ⊥ - χ||)]0.5 [6], where D, and χ⊥ (χ||) represent the anisotropy energy, and the magnetic susceptibility along the hard axis (easy axis), respectively. Assuming χ⊥ to be the susceptibility value just above the AF transition temperature, χ⊥ ~ 3.8 mJT-2mol-1 for the Me4P salt, and 2.7 mJT-2mol-1 for the Me4Sb salt [7], the D value can be estimated to be 9.2 mJmol-1, and 1.6 mJmol-1, respectively; the anisotropy energy of the Me4Sb salt is much lower than that of the Me4P salt. This is a reason why the spin-flop transition is obscured in the SQUID measurements on the Me4Sb salt.
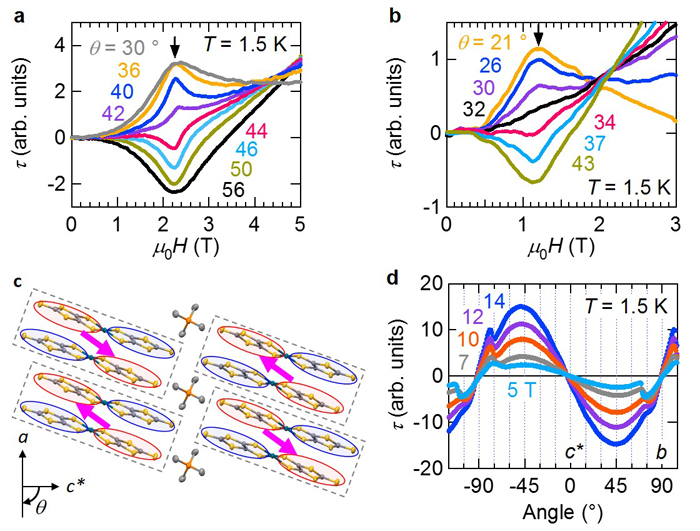
Next, we discuss the orientations of the easy axis, θ ~ 43 ° for the Me4P salt and θ ~ 32 ° for the Me4Sb salt, which quite deviate from the a and c* axes. The recent theoretical study predicts that there is a nonuniform spin-density distribution in a Pd(dmit)2 molecule [8], as shown in the ellipsoids in Fig. 1c. The orientation of the easy axis determined in the present work well agrees with the direction which connects the high-density parts (two red ellipsoids) in a dimer, [Pd(dmit)2]2-. When the magnetic field is applied parallel to the bc* plane, a characteristic kink of the magnetic torque is observed near the b axis in the wide field range up to 14T for both the salts, as shown in Fig. 1d. Such torque anomalies are not expected for a simple uniaxial antiferromagnet. Further experiments will be required to understand what happens when H // b.
References
[1] R. Kato, Bull. Chem. Soc. Jpn., 87, 355 (2014).
[2] D. Watanabe et al., Nat. Commun., 3, 1090 (2012).
[3] M. Yamashita et al., Science, 328, 1246 (2010).
[4] K. Ueda et al., 69th JPS meeting, 27aBC-1 (2014).
[5] T. Kawamoto et al., Phys. Rev. B, 77, 224506 (2008).
[6] K. Yoshida et al., Prog. Theor. Phys., 6, 691 (1951).
[7] M. Tamura et al., J. Phys. Condens. Matter., 14, L729 (2002).
[8] H. Seo et al., J. Phys. Soc. Jpn., 84, 044716 (2015).
Assign: Oshima, Kim, Kato
The anion radical salts β'-X[Pd(dmit)2]2, where X is a monovalent cation and the space group is C2/c, are Mott insulators which consist of S=1/2 spins on a triangular lattice [1]. These salts have attracted attention over the years since spin-frustration parameters t'/t can be controlled by substituting the cation X as shown in Fig. 1. The X=Me4P salt has a highest Néel temperature TN=40 K with t'/t close to 0.6. Then, TN decreases as t'/t getting closer to an isotropic triangle (t'/t ~ 1) where TN for X=Et2Me2P and Me4Sb salts are 14 K and 11 K, respectively. In contrast, the X=EtMe3Sb salt, where t'/t ~ 0.9, shows no long-range order down to 30 mK. It is considered that a gapless quantum spin-liquid (QSL) state is achieved in this compound [2-4].
To understand more deeply the phase diagram and investigate the spin correlations and the spin dynamics of the β'-X[Pd(dmit)2]2 system, we have performed in-plane ESR measurements on X=Me4P, Et2Me2P, Me4Sb and EtMe3Sb salts. The β'-X[Pd(dmit)2]2 system contains two layers of triangular lattice in the unit cell, where the stacking direction of the Pd(dmit)2 dimers are different for each layer known as a solid crossing column structure (Fig.1 left). These layers are crystallographically equivalent, however g-tensor for each layer is different since the stacking direction of the dimers are different. Reflecting the solid crossing column structure, the in-plane angular dependence of the ESR spectra for the X=EtMe3Sb salt shows two ESR absorptions coming from two different anion layers. Meanwhile, only a single absorption, due to the exchange narrowing, is observed for the X=Me4P salt (TN=40 K). These results suggest the interlayer exchange interaction J is infinitesimal, estimated ca. 0.37 mK, for the X=EtMe3Sb salt and finite for the X=Me4P salt. Two absorption lines were observed also for the X=Me4Sb salt (TN=11 K) where J is estimated around 1.58 mK. For the X=Et2Me2Sb salt (TN=14 K), J > 76 mK is obtained. Hence, it is found that TN is related with the interlayer exchange interaction. Meanwhile, the ESR linewidth of X=EtMe3Sb shows a gradual narrowing with decreasing temperature (Fig. 2 top). Since ESR transition does not occur for a singlet spin state, ESR should probe the elementary spin excitation of the QSL state, known as a spinon. We suppose the linewidth narrowing is due to the dynamics of the elementary excitation (motional narrowing). Next, the power dependence of the ESR intensity for the X=EtMe3Sb salt is studied (Fig.2 bottom). ESR intensity usually saturates by increasing the power of the microwave. This saturation phenomenon occurs because the energy levels, where the ESR transition occurs, become equally populated by high microwave power. Such phenomenon is frequently observed at low temperature due to the long spin-lattice relaxation time. As shown at the bottom of Fig. 2, the ESR intensity does not saturate up to 200 mW, and keeps the linear relation with P1/2, which is proportional to the intensity of the microwave. We suppose that the saturation was not observed since the ground state is highly degenerated and short relaxation time is expected for the QSL state. Owing to the observation of such peculiar ESR behaviors, we conclude that ESR is directly probing the spinons in the QSL state.
Finally, we report the ESR results for EtMe3P[Pd(dmit)2]2 (space group P21/m). Although this salt also consists of a triangular lattice of S=1/2 spins, its crystal structure is different from that of the '-X[Pd(dmit)2]2 salts. Instead of having the solid crossing column structure, EtMe3P[Pd(dmit)2]2 takes the parallel column structure as schematically shown at the top right of Fig. 1. This salt turns into a valence bond solid (VBS) state at TVBS=25 K. The spin situated at the corner of the triangular lattice makes a singlet pair with its nearest neighbor spin, and the system becomes non-magnetic. It is interesting that EtMe3P[Pd(dmit)2]2 is different from the β'-X[Pd(dmit)2]2 in the ground state. Therefore, we have microscopically investigated the electronic state of EtMe3P[Pd(dmit)2]2 with in-plane ESR.
The angular dependence of the g-value for EtMe3P[Pd(dmit)2]2 is similar to that for the β'-type salts, where the g-tensor is related to the stacking direction of the Pd(dmit)2 dimers. Only a single ESR absorption is observed for EtMe3P[Pd(dmit)2]2 owing to the parallel column structure. The temperature dependence of the ESR's integrated intensity is shown in Fig. 3. The integrated intensity starts to decrease below 50 K, and the contribution of the spins is minimized below TVBS. Then, Curie component of the residual spins is observed at the lowest temperature. The residual spins are about 0.1 % of the total spins. We considered a model where the single spin situated at each corner of the triangular lattice makes a singlet pair with its neighbor, and gradually decreases with exp(-ΔE/kBT). Then, the excited state of the singlet state, which increases with exp(-ΔE/kBT), also contributes to the ESR intensity. We have successfully fit our result with this model when ΔE=135 K. To confirm our model, high-field ESR measurement is planned to study the field dependence of the spin gap ΔE.

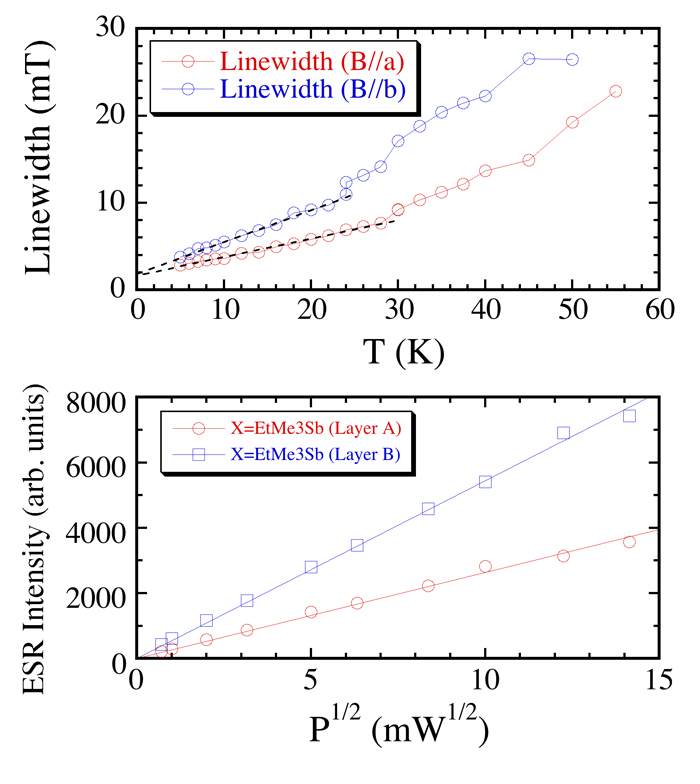

Figure 3: Temperature dependence of the ESR's integrated intensity for EtMe3P[Pd(dmit)2]2
[1] R. Kato, Bull. Chem. Soc. Jpn., 87, 355 (2014).
[2] S. Yamashita et al., Nature Commun., 2, 275 (2011).
[3] M. Yamashita et al., Science, 328,1246 (2010).
[4] T. Itou et al., Nature Phys., 6, 673 (2010).
[5] M. Tamura et al., J. Phys. Soc. Jpn., 75, 093701 (2006).
Assign: Fujiya,am Kato
Spin-orbit coupling (SOC) is a key concept in modern condensed matter physics, which is argued to stabilize topological insulators, Wyle semimetals, and spin transport phenomena. So far, molecular conductors that provide a playground of correlated electrons have been considered to be nothing to do with the SOC related physics, and indeed, the g-factors of most organic conductors are close to two.
A series of (Cation)[Pt(dmit)2]2 salts are related to Pd(dmit)2 salts including a quantum spin liquid candidate (Fig. 1). The resistivity of the Me4P salt shows a metal-insulator transition at T~200K (Fig. 2). The measurement of the uniform susceptibility shows an abrupt disappearance below the transition temperature. They are why the nature of the transition is a metal-to-band insulating transition caused by the structural change. Apart from this early days understanding of the phase transition, there remains an open question in the electronic correlation in the metallic state. The susceptibility is much different from conventional Pauli paramagnetism but decreases in lowering temperatures.
To resolve the problem, we performed 13C NMR measurements on (Cation)[Pt(dmit)2]2. The nuclear spin-lattice relaxation rate (1/T1) shows an abrupt decrease just below the transition temperature with a large energy gap with ~3000K, which supports the band insulating ground state (Fig. 3). On the other hand, 1/T1 for T>220K are nearly independent of temperature, which we can usually expect for antiferromagnetic insulators. We can consider the bulk electronic state of (Cation)[Pt(dmit)2]2 salts is a Mott insulator, or a highly correlated electronic system. We estimate the antiferromagnetic coupling constant, J, as large as several hundred kelvins like that of well established Mott insulator system, the Pd(dmit)2 salts. We calculated the band structure taking the spin-orbit coupling particularly induced by the 5d transition metal, Pt, and found that the SOC provides a single band at EF (Fig. 4). This single band could be a source of the half-filled Mott insulating state.
This study has revealed that the spin-orbit coupling plays a striking role for the electronic correlation even in the organic conductors. The spin-orbit coupling set by the central Pt ion narrows the bandwidth establishing a single band, and the Coulomb repulsion in the electrons can cause the system insulating.

Figure 1: Molecular structure of Pt(dmit)2. The central white circle is Pt ion.
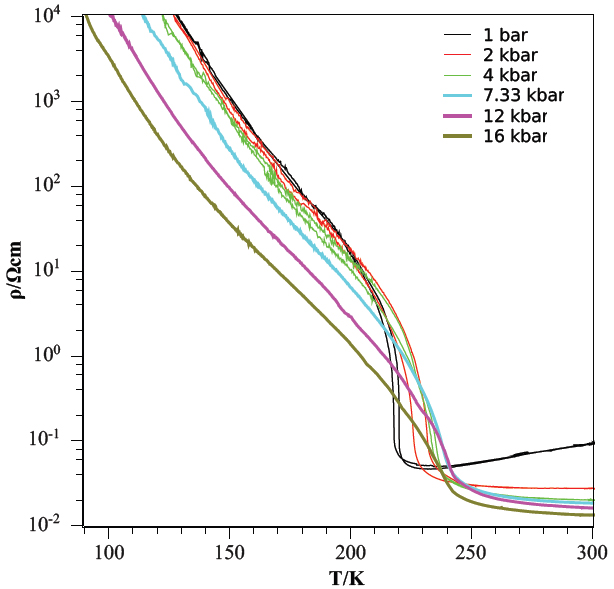
Figure2: Resistivities of Me4P[Pt(dmit)2] (Abdel-Jawad, Nomura)


Figure 4: Band calculations of the high-temperature state of Me4P[Pt(dmit)2]2 using Quantum-Espresso package.
The left panel shows the dispersion relation neglecting the spin-orbit coupling, and the right shows that including SOC.
[1] T. Ishikawa et al., Science, 350, 1501 (2015).
Assign: Cui, Kawasugi, Kato; Tsumuraya
Since the discovery of the first single-component molecular metal [Ni(tmdt)2] (tmdt = trimethylenetetrathiafulvalenedithiolate), investigation of single-component molecular metals based on metal dithiolen complexes has attracted considerable attention.[1] However, due to the difficulty of growing a single crystal, ambient-pressure metallic state has been found only in [M(tmdt)2][2] up to now. On the other hand, molecular crystals have a soft lattice, thus an application of pressure to insulating single-component molecular crystals is very efficient way to discover new single-component molecular metals or superconductors. Indeed, we modified the four-probe resistivity measurement technique by using a Diamond Anvil Cell (DAC), and successfully found that insulating [Ni(ptdt)2] (ptdt = propylenedithiotetrathiafulvalenedithiolate) [3], [Cu(dmdt)2] (dmdt = dimethyltetrathiafulvalenedithiolate) [4], and [Ni(dmit)2] (dmit = 1,3-dithiole-2-thione-4,5-dithiolate) [5] turned metallic at 19.4 GPa, 4.7 GPa, and 15.9 GPa, respectively. Recently, we also found the first single-component molecular superconductor based on metal dithiolene complex [Ni(hfdt)2] (hfdt = bis(trifluoromethyl)tetrathiafulvalenedithiolate) (Tc= 5.5 K at 8 GPa) [6]. Very recently, we have synthesized two type of single crystals(type I and type II)of [Pt(dddt)2] (dddt=5,6-dihydro-1,4-dithiin-2,3-dithiolate), and found that the Type II [Pt(dddt)2] shows a complete metallic state down to 2K at 9.6 GPa.
Single crystals of [Pt(dddt)2] were prepared from [(n-C4H9)4N]2[Pt(dddt)2] by I2 oxidation. As shown in Fig. 1, the block shaped Type I crystal is isostructural with [Pd(dddt)2] which emerges Dirac cones at 8 GPa [7]. In Type II crystal, [Pt(dddt)2] molecules weakly dimerize and form a κ-type like structure, and each dimers are stacking along the side-by-side direction. A modified DAC four-probe DC method was used for all measurements. Tension annealed SUS301 was used as the gasket, and Daphne Oil 7373 was used as the pressure transmitting medium. Electrical contacts were obtained by attaching four 10 μm gold wires with gold paint. The pressure was determined by the shift of the ruby fluorescence R1 lines at room temperature.
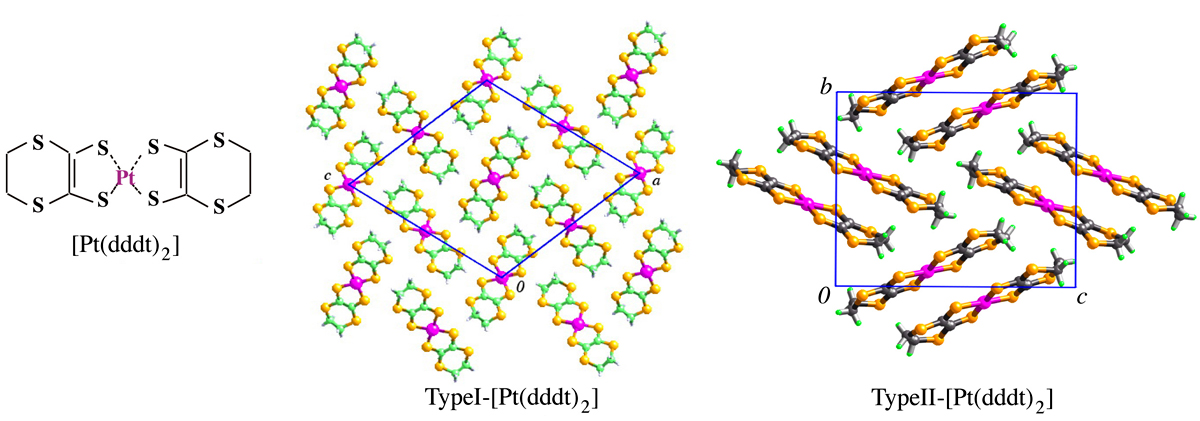
Figure1: Molecular and crystal structure of [Pt(dddt)2]
As shown in Fig. 2, the Type I [Pt(dddt)2] at 5.7 GPa showed the room temperature conductivity(σ) 4 x 10-4 S cm-1, and excitation energy (Ea) 0.23 eV. These values are similar to those for [Pd(dddt)2] at 4.2 GPa (&sugma; = 5 x 10-4 Scm-1, Ea = 0.2eV) . Under pressures lower than 8.5 GPa, the temperature dependence of resistivity was similar to the result obtained from [Pd(dddt)2]. In contrast to that [Pd(dddt)2] crystal exhibits temperature independent resistivity at 12.6 GPa, the [Pt(dddt)2] crystal exhibits a semiconducting behavior with Ea = 17 meV, at 13.3 GPa (Fig. 2). However, a sharp drop of room temperature resistivity occurred between 6.5 GPa and 8.5 GPa. This kind of big change was often associated with structure changes, and we need to analyze high pressure structure to verify it. Resistivity of Type II [Pt(dddt)2] crystal was measurable from 2.6 GPa. The room temperature resistivity and Ea gradually decreases with increasing pressure. At 9.6 GPa, the system turned to a complete metallic state down 2 K (Fig. 3). The ab initio DFT calculation was performed for high pressure band structure of Type II [Pt(dddt)2]. A metallic band structure was obtained at 10 GPa, which well explains experimental results obtained by our DAC measurements.
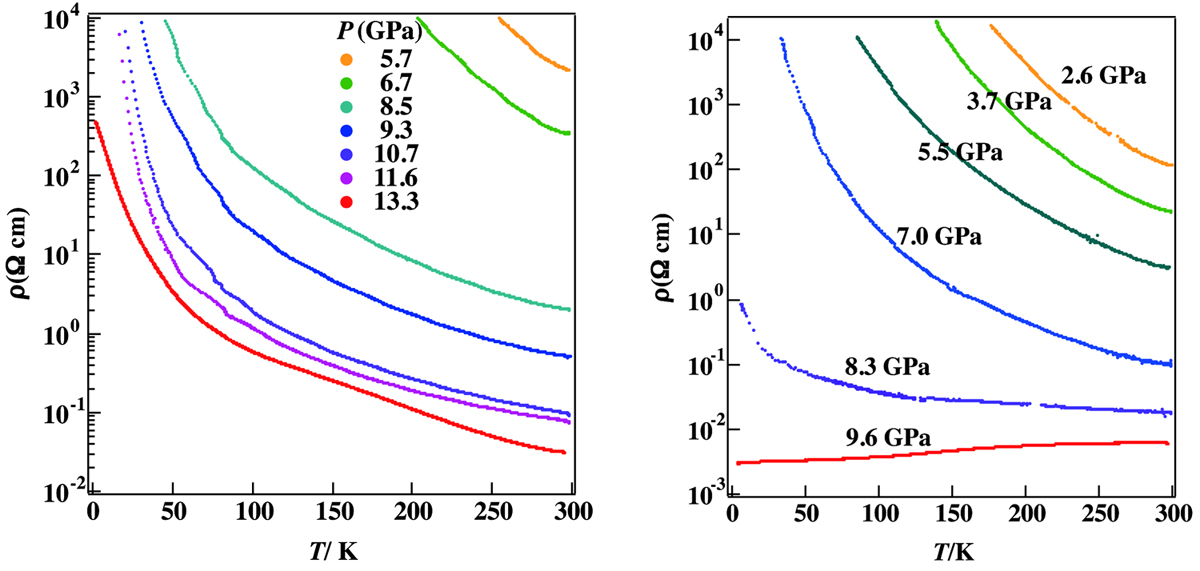
Figure 2: Temperature dependence of resistivity for Type I [Pt(dddt)2] under high pressure.
Figure 3: Temperature dependence of resistivity for Type II [Pt(dddt)2] under high pressure.
References
[1] H. Tanaka, Y. Okano, H. Kobayashi, W. Suzuki, A. Kobayashi, Science, 291, 285 (2001).
[2] W. Suzuki, E. Fujiwara, A. Kobayashi,Y. Fujishiro, E. Nishibori, M. Takata, M. Sakata, H. Fujiwara, H. Kobayashi, J. Am. Chem. Soc., 125, 1486 (2003).
[3] H. B. Cui, J. S. Brooks, A. Kobayashi, H. Kobayashi, J. Am. Chem. Soc., 131, 6358 (2009).
[4] B. Zhou, Y. Idobata, A. Kobayashi, H. B. Cui, R. Kato, R. Takagi, K. Miyagawa, K. Kanoda, J. Am. Chem. Soc., 134, 12724 (2012).
[5] H. B. Cui, T. Tsumuraya, T. Miyazaki, Y Okano, R. Kato, Eur. J. Inorg. Chem., 24, 3837 2014).
[6] H. B. Cui, H. Kobayashi, S. Ishibashi, M. Sasa, F. Iwase, R. Kato, A. Kobayashi, J. Am. Chem. Soc., 136, 7619 (2014).
[7] R. Kato, H. B. Cui, T. Tsumuraya, T. Miyazaki, Y. Suzumura, J. Am. Chem. Soc., inpress.
Assign: Kawasugi, Sato, Kato; Seki, Edagawa, Pu, Takenobu, Yunoki, Yamamoto
In materials with a half-filled band, strong onsite Coulomb repulsion localizes the conduction electron at each site. This type of insulator is called a Mott insulator. When electrons or holes are doped into a Mott insulator, the commensurability between the electron density and the lattice potential is reduced, and the electrons are delocalized (band-filling-controlled Mott transition). The Mott transition has been intensively studied because exotic phenomena such as pseudogap and high-temperature superconductivity emerge near the transition point in some cuprates.
Among molecular conductors consisting of organic molecules, there are some material groups in which a superconducting phase borders the Mott insulating phase. Common nature with the high-temperature superconductors has been discussed on those materials [1]. To provide insights into the universal nature of the Mott transition, it is also important to investigate the doping effect in those organic Mott insulators. However, chemical doping by element substitution has been limited in molecular conductors because of the difficulty of synthesis. We have succeeded in carrier doping into an organic Mott insulator κ-(BEDT-TTF)2Cu[N(CN)2]Cl (κ-Cl hereafter) using an alternative doping method, electric-double-layer transistor (EDLT). This method enabled reversible and high-density (more than 20%) doping in the same sample (Fig. 1).

According to our measurements of the transport properties and calculations of the single-particle spectral function of the Hubbard model, the effect of carrier doping into the organic Mott insulator is summarized as follows. The band filling deviates from 1/2 by either electron or hole doping, resulting in emergence of the Fermi surface. However, reflecting the non-interacting band structure, pseudogap opens at specific points in the momentum space. A major pseudogap opens near the van Hove critical point only under hole doping. Namely, the effect of doping is quite asymmetric.
This year we investigated the doping dependence of the thermoelectric properties. According to the Mott formula, the Seebeck coefficient of metals or degenerate semiconductors is proportional to the inverse density of states (1/N) and the energy gradient of the density of states (∂N/∂E) at EF. Therefore, large S is expected in the pseudogap state that has a dip of N near EF. In addition, the general trade-off relation between the conductivity and the Seebeck coefficient is possibly violated due to large enhancement of ∂N/∂E, resulting in high thermoelectric power factor.
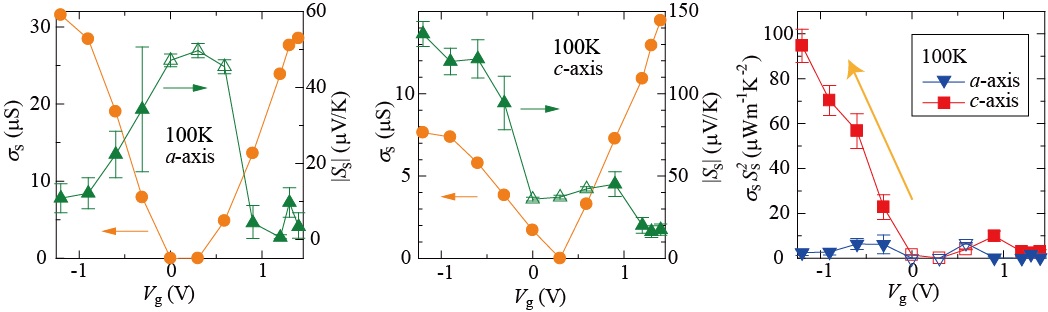
To test this, we measured the gate voltage dependence of the conductivity σ, Seebeck coefficient S, and power factor σS2 in the κ-Cl EDLT. According to the band structure, the effect of the pseudogap appears strongly along the crystallographic c-axis. The sample was therefore shaped into a cross (Fig. 2) and the measurements were performed along the two in-plane axes (a and c). This sample was insulating at low temperature even under doping, probably because of random potential at the surface. However, metallic conductivity (dσ/dT < 0) was observed down to about 100K, indicating that the Mott transition occurs by both electron and hole doping at high temperature.

Figure 3 shows the gate voltage dependence of σ, S, and σS2 at 100K. While S along the a-axis was decreased by electron or hole doping, the value along the c-axis was increased by hole doping. This is in contrast to the general trade-off relation between the conductivity and the Seebeck coefficient, resulting in relatively high power factor for an organic material. Although the value is less than those of optimally doped conducting polymers, these results indicate that the pseudogap in strongly correlated electron systems can be applied to improve thermoelectric properties of a material.
References
[1] R. McKenzie, Science, 278, 820 (1997).
[2] Y. Kawasugi et al., Nat. Commun., 7, 12356 (2016).
[3] Y. Kawasugi et al., Appl. Phys. Lett., 109, 233301 (2016).
Assign: Sato, Kawasugi, Kato; Yamamoto
Recent advances in thin-layer fabrication techniques have promoted a growing interest in the low-dimensional electron systems. Among them, particularly intriguing are materials with strongly correlated electrons because they exhibit a wide variety of exotic phases such as Mott insulators, superconductors, and spin liquids. Central issues are the understanding of fundamental physics underlying these phenomena and the establishment of controlling the phase transitions. Focusing on the doping-driven metal-insulator transition on Mott insulators (filling-controlled Mott transition), we investigate the carrier transport properties of a solid-gate-type field-effect transistor (FET) using a single crystal of κ-(BEDT-TTF)2[Cu(CN)2]Cl (κ-Cl, where bis(ethylenedithiotetrathiafulvalene) is referred to as BEDT-TTF), a typical quasi-two-dimensional molecular Mott insulator. Because of the advantages of field-effect doping (e.g., continuous, reproducible, clean (without introducing chemical impurities), and real-time control of doping is possible even at low T ), we can systematically investigate the characteristics of the transition, which are obscured in the previous studies using conventional doping methods. Very recently, improving the FET channel interface, we found quantum criticality of the conductivity in the metal-insulator crossover regime at middle T (T>8 K). We describe here the lower-T measurement focusing on the "disorder"-induced effects to elucidate the origin of the quantum criticality.

Figure 1D shows the low-T magnetoresistance (MR) for perpendicular magnetic field. Corresponding to metal-insulator transition at ρ~e2/h, the MR rapidly changes with a sign inversion. Measurements for the angle-dependent MR using a superconducting split magnet reveals that the sign inversion of MR stems from imbalance of two competing components: the one is spin-origin, showing an isotropic and positive MR, and the other is orbital-origin, showing an anisotropic and negative MR that depends only on the perpendicular component of magnetic field, B⊥. As shown in Figure 2B, the field-angle-dependence of MR is described by the Hikami-Larkin-Nagaoka formula [2]:

where Ψ, l, Lφ are the digamma function, the mean-free path, and the decoherence length, respectively. Hence, the anisotropic MR component is assigned to weak localization effect. From systematic investigation on the weak localization component for various T and Vg, one can indentify a clear 1/√T-law for coherence length, which supports the "disordered Fermi liquid" scenario in the low-T metal regime. The decoherence length dependent on Vg (Figure 2E) seems tied to modification of quasiparticle lifetime owing to the enhancement of the correlation near the transition point.
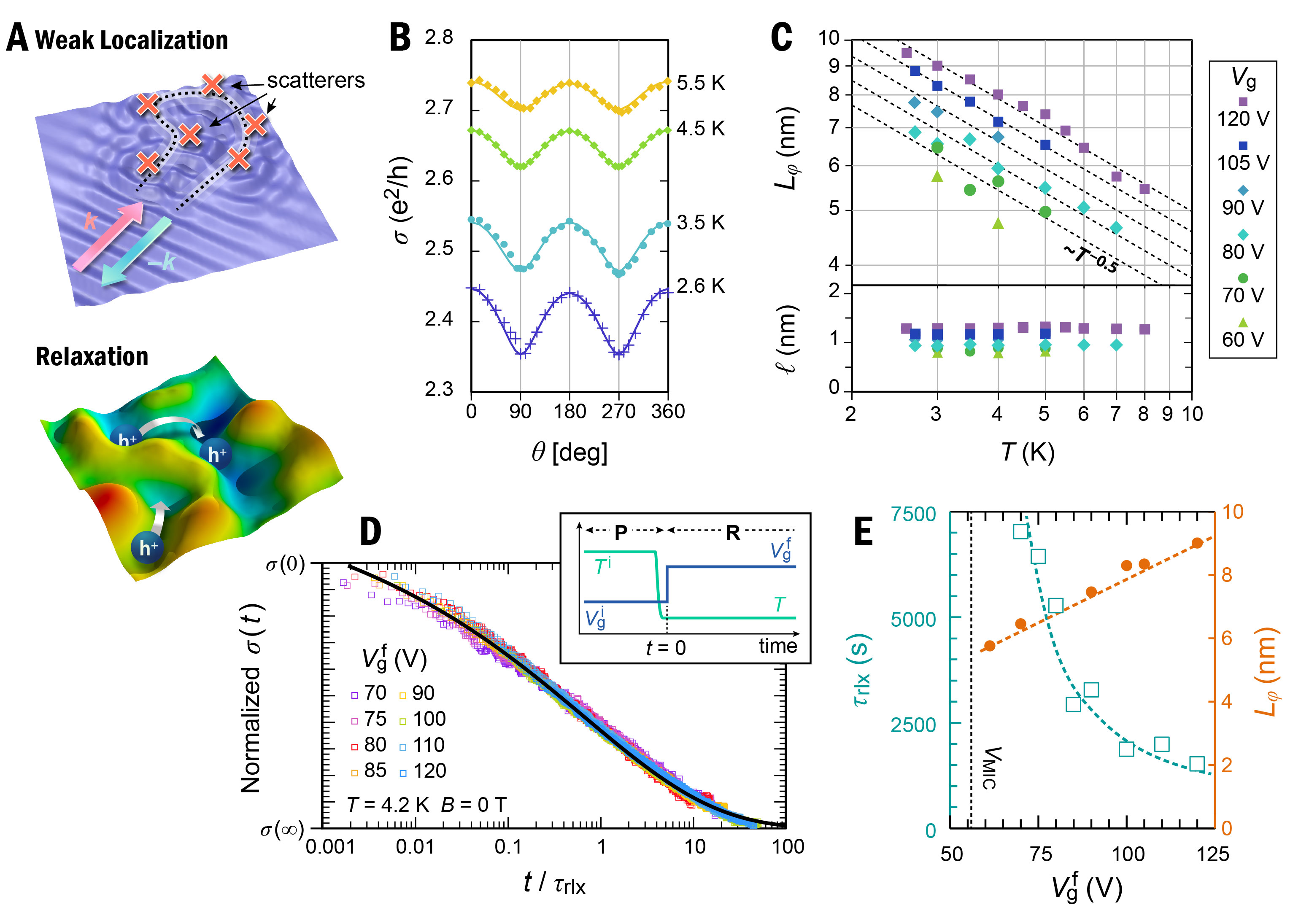
Next we present the systematic measurement of the conductivity relaxation as an example of the disorder/inhomogeneity effects. Here, "relaxation" denotes the long-period temporal change in conductivity after a modulation of the external field at low T. Figure 2D shows the relaxation curves of conductivity obtained after a rapid change in the filling from Vg=0 V to various voltages. Each curve can be scaled to the nonexponential Ogielski relaxation (a hybrid formula combining the short-t power law and the long-t stretched exponential law[3]), which indicates that the system becomes glassy in the presence of disorders. The characteristic relaxation time (τrlx) drastically increases in the vicinity of the transition point (Figure 2E), representing enhancement of the energy scale for relaxation by the strong electron correlation. These results for the weak localization and the conductivity relaxation consistently manifest the remarkable evolution of the electron correlation in the narrow doping window of Δp~0.02 around the Mott transition. Our study will provide an impetus for theoretical investigation of Mott transition, especially regarding the microscopic description of the low-T phases.
References
[1] Y. Sato et al., Nano Lett. (in press)
[2] S. Hikami, A. L. Larkin, Y. Nagaoka, Prog. Theor. Phys., 63, 707 (1980).
[3] A. T. Ogielski, Phys. Rev. B, 32, 7384 (1985).
Assign: Oshima, Lee, Cui, Kato
Molecular conductors with finite π-d interaction have attracted considerable interests for the past few decades since interaction between the itinerant π-electron and localized d-electron yields some intriguing physical phenomena. λ-(BETS)2FeCl4 is one of the such materials which shows interesting physical properties. Although it is well known that this salt shows superconducting state above 17 T when the magnetic field is applied along the conducting plane [1], the ground state at low temperature is also interesting. This salt shows a metal-insulator transition, and becomes simultaneously antiferromagnetic at TN=8.3 K known as the antiferromagnetic insulating (AFI) phase. The origin of the antiferromagnetic state, whether the d-electrons or the π-electrons trigger the antiferromagnetic state, is discussed for a long time. However, recent specific heat measurements revealed that the broad excess specific heat observed below TN can be fitted with the Schottky peak in which the six energy levels of Fe3+ (S=5/2) is split by an internal field of 4 T. Therefore, it is considered that only -electrons become antiferromagnetic (inducing an internal field of 4 T), and the d-electrons of Fe3+ remain paramagnetic. [2] Recently, Mössbauer and magnetic torque measurements supported this paramagnetic Fe scenario. However, previous ESR studies did not observe electron paramagnetic resonance (EPR) in the AFI phase, and only antiferromagnetic resonance (AFMR) was observed. [5-7] Moreover, anomalous dielectric constant and anomalous high-frequency response within the AFI phase are previously reported, and its origin remains unclear. [7,8] Hence, to resolve these issues, we have performed ESR measurements of λ-(BETS)2FeCl4 in detail.
Figure 1 shows the angular dependence of g-value and ESR linewidth obtained from EPR above TN. The magnetic field was rotated perpendicular to the c-axis. Note that the conducting plane is the ac-plane. Single EPR line was observed in the whole temperature range. This suggests that the signals from π- and d-electrons are merged to a single EPR line due to the exchange narrowing. On the other hand, the g-value shows a peculiar angular dependence (see Fig. 1 top). In general, the g-value shows the (1+cos2θ) dependence, however, the angular dependence of λ-(BETS)2FeCl4 shows two dips around 30° and 90°, which become distinct by decreasing temperature. These two angles approximately correspond to the direction where two short bond lengths between S(Se) of BETS molecule and Cl of the FeCl4 exist. Therefore, this peculiar angular dependence is due to the π-d interaction of the system. Below 10 K, EPR shifts and linewidth broadening occurs, which are the typical EPR behaviors above TN. Then, the EPR disappears around TN, and AFMR is starting to be observed along the direction of the easy-axis. These behaviors are consistent with previous studies. [5]。

Figure 1: Angular dependence of the g-value (top) and ESR linewidth (bottom).
The magnetic field is rotated perpendicular to the c*-axis.
In summary, our results suggest (i) π-d interaction of the system is strong in the whole temperature range since only a single EPR line is observed, (ii) the d-electrons mainly contribute to the magnetic properties in the high temperature region since the π-electrons are itinerant, however, the π-electrons affect the magnetic properties at low temperature. (This is why two minima in the g-value are observed) And (iii) partial antiferromagnetic long-range order occurs above TN, and the easy-axis is tilting as temperature decreases in the AFI phase. Namely, the d-electrons mainly contribute to the magnetism of λ-(BETS)2FeCl4, but the contribution of π-electrons increases as temperature decreases. In turn, the orientation change of the easy-axis by temperature also suggests that the π-electrons are not fully localized. Actually, we have previously observed that the resistance is still gradually increasing below TN, and dielectric anomaly and high-frequency response are previously reported as mentioned above. [7,8] All these results suggest that the π-electrons are not fully localized below TN. We expect that this slow localization of π-electrons is affecting the magnetic properties of the system. High-field ESR measurements are planned to investigate the electronic state of the AFI phase in the high-field region.

Figure 2: Angular dependence of the AFMR's resonance field.
[1] S. Uji, et al., Nature, 410, 908(2001).
[2] H. Akiba, et al., J. Phys. Soc. Jpn., 78, 033601 (2009).
[3] J. C. Waerenborgh, et al., Phys. Rev. B, 81, 060413 (2010).
[4] S. Sugiura, et al., J. Phys. Soc. Jpn., 85, 064703 (2016).
[5] L. Brossard, et al., Eur. Phys. B, 1, 439 (1998).
[6] T. Suzuki, et al., Phys. Rev. B, 67, 020408(R) (2003).
[7] I. Rutel et al., Phys. Rev. B, 68, 144435 (2003).
[8] E. Negishi et al., Phys. Rev. B, 71, 012416 (2005).
Assign: Kawasugi, Kato; Tajima, Suda, H. Yamamoto
A multilayered massless Dirac fermion system, α-(BEDT-TTF)2I3 under high pressure, provides the testing ground for the investigation of physical phenomena in strongly correlated Dirac particles. This massless Dirac fermion phase is close to the charge ordered insulating (CO) phase on the pressure-temperature phase diagram. In this work, the quantum transport phenomena in the thin crystals on the PEN substrate were investigated at the vicinity of the phase boundary. We revealed that the CO phase close to the boundary was in the "massive" Dirac fermion systems. It strongly suggests that we can control the mass of Dirac particles by the pressure. Moreover, in this state, massive Dirac particles and normal particles coexist.
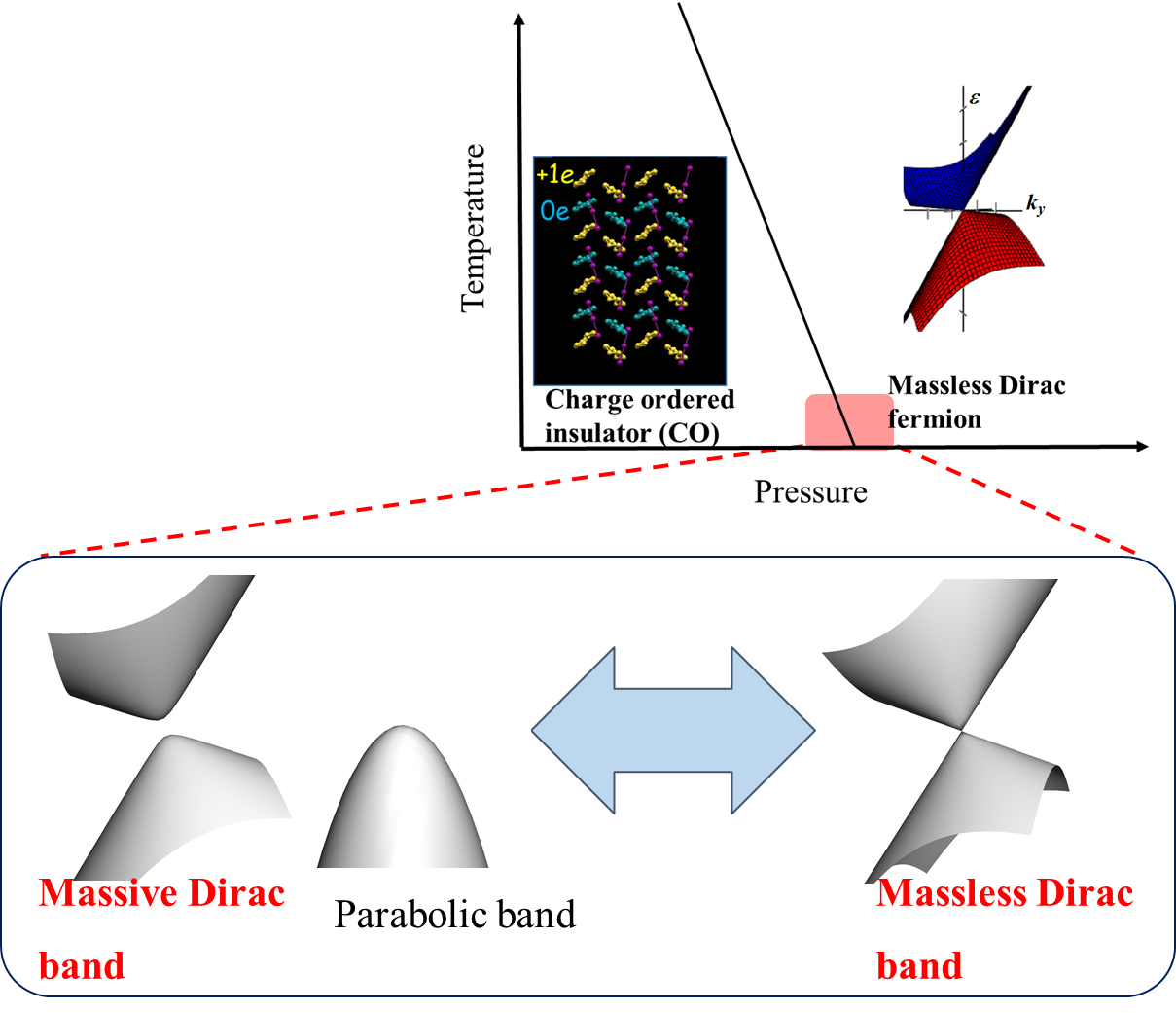
Figure : Schematic band structure at the vicinity of phase boundary
Assign: Abdel-J, Kato; Watanabe, Tajima, Ishii
The metal-insulator transition of the Mott type can be achieved in two ways. Filling-controlled Mott transitions are achieved by adding charge carriers through chemical doping or electric field while bandwidth-controlled Mott transitions require the control of the ratio U/W between the on-site Coulomb repulsion, U and the bandwidth, W. Bandwidth-controlled Mott transitions can be achieved by chemical pressure or, as in this study, by physical pressure in various media. By performing pressure sweep measurements of the conductivity, &sugma; at constant temperature (isothermal) in (V1-xCrx)2O3, Limelette et al.[1] found that σ on the metallic side of a three-dimensional Mott insulator could be scaled. From those scalings, they extracted critical exponents of the transition which were consistent with Ising universality class. The same measurement was subsequently performed in κ-(BEDT-TTF)2Cu[N(CN)2]Cl [2] a quasi-two-dimensional (quasi-2D) organic conductor, where BEDT-TTF stands for bis(ethylenedithio)tetrathiafulvalene. This subsequent study showed that although the conductivity on the metallic side of the phase diagram could still be scaled around the critical endpoint; the extracted critical exponents were inconsistent with any of the known universality class. NMR data in the same quasi-2D compound [3] further support those anomalous critical exponents while thermal expansion [4] measurements in the same class of compounds find critical exponents that correspond to mean field theory. Theoretical interpretations of those results are either in support of the unconventional critical exponents values or against.
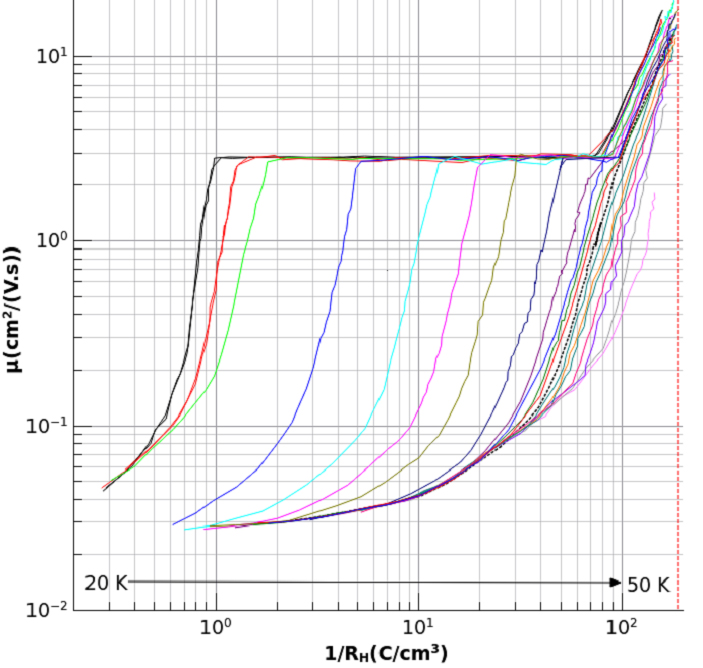
To resolve this issue, we have performed isothermal pressure measurement, with helium as pressure medium, of the conductivity, σ, thermoelectric power coefficient, SB and the Hall coefficient, RH in Pd(dmit)2 based compounds through the Mott transition. Figure 1 shows the results of the isothermal pressure dependence of σ and RH in EtMe3P[Pd(dmit)2]2 a quasi-2D organic conductor. The Mott transition is clearly seen through the RH measurement, with a 1st order phase transition jump at temperatures below the critical temperature of 30 K. In the metal state, RH saturates to a value of 0.0053cm3/C (red dotted line), equivalent to 1 charge per dimer site, as expected from a half-filled state. If we assume that throughout the Mott transition RH=1/ne, where n is the carrier number and e is the electron charge; then we can define the carrier mobility, μ from the relation σ = neμ. Fig. 1 shows the isothermal pressure dependence of μ through the Mott transition. Interestingly a plateau behavior of μ is visible with a value which appears to be related to the Mott-Ioffe-Regel limit. The Mott-Ioffe-Regel limit states that in a metallic state, the mean free path distance of a carrier jump must be greater or equal to the unit cell distance. Figure 2 shows that this plateau behavior of μ is further accentuated by plotting μ with respect to the carrier number (i.e. inverse RH) and that it is related to the 1st order phase transition.
Measurements of the isothermal thermoelectric power coefficient, SB, in EtMe3P[Pd(dmit)2]2, exhibits a sharp minimum in its pressure dependence at the Mott transition[5]. Using this minimum of SB, we were able to determine objectively the pressure and temperature limit of the Mott transition. By reinterpreting the relation between σ and the scaling exponents[5], We were able to give a consistent scaling of σ and SB to a 2D Ising universality class from both measurements.
Comparing the results of the Hall coefficient and the thermoelectric power reveals that each probes have distinctive advantages. For instance, RH values drastically change at the insulating limit of the 1st order phase transition but do not change much at the metal limit; while inversely, SB can delimit, with ease, the metal limit of the 1st order phase transition.
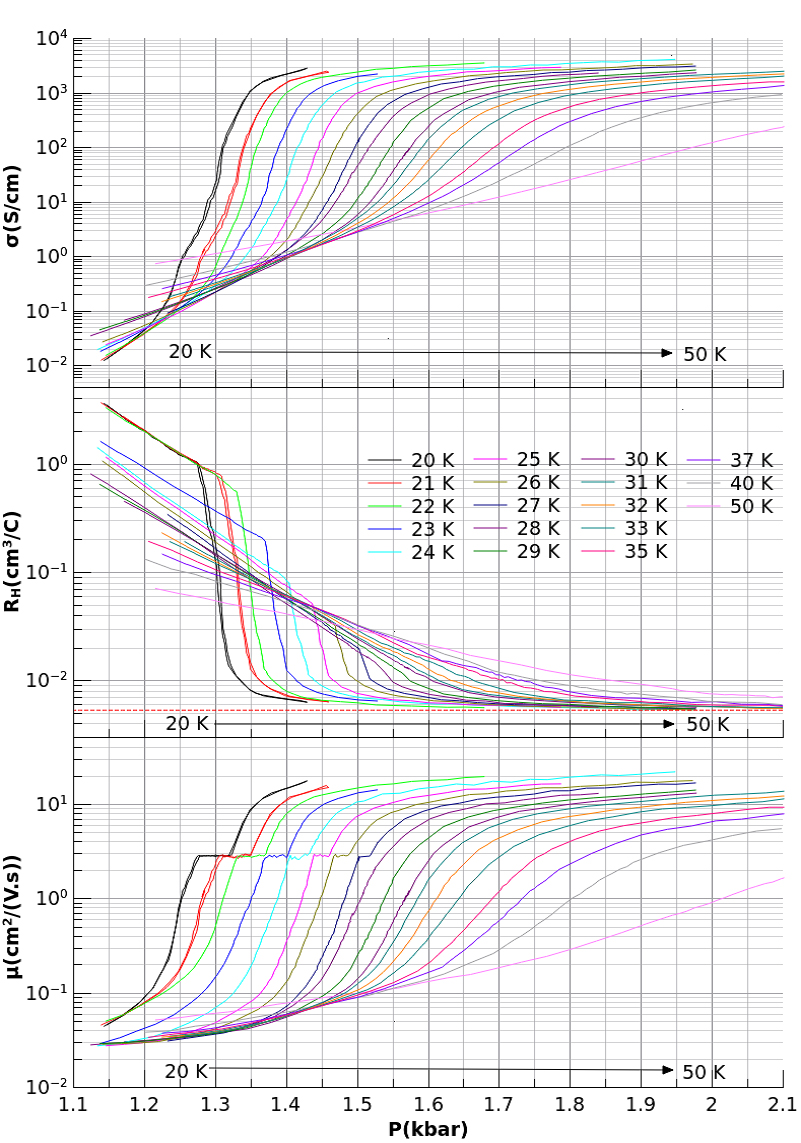
Figure 1: Isothermal pressure dependence of the conductivity, Hall coefficient and charge mobility in EtMe3P[Pd(dmit)2]2.